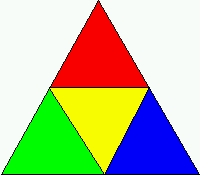
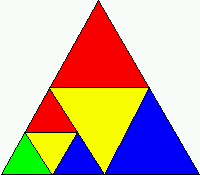
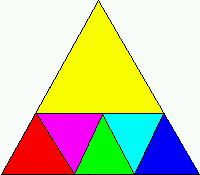

The largest number of equilateral triangles that cannot be obtained by cutting an equilateral triangle into pieces is 5.
| Four pieces (2 or 3 are not possible). Any of the
four pieces can be further divided in the same |
 |
 |
... to obtain a total of seven pieces. This process can be followed to get 10, 13, 16, 19, ... pieces. |
| Six pieces. Any of the six pieces can be further divided into four more pieces, and so on, to yield 9, 12, 15, 18, ... pieces. |  |
 |
Eight pieces. Again, dividing any of these into four more pieces allows for 11, 14, 17, 20, ... pieces. |
It's easy to see that any number of pieces greater than five is possible by combining the strategies above. The only impossible numbers of pieces are 2, 3, or 5.
Remarkably, if you begin with a square and cut it into squares, the answer is the same (any number of pieces other than 2, 3, or 5 is possible). Can you prove this?
After seeing these two examples, you might be tempted to believe that any shape that can be cut into smaller pieces of the same shape would share this property with equilateral triangles and squares, but this hypothesis is incorrect. Can you think of a shape that can be cut into any number of smaller pieces of the same shape?