
Here is a twist on an old puzzle:
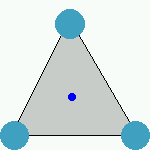
You have sixteen coins. One of them is a counterfeit, and the other fifteen are genuine, but identical in appearance to the counterfeit. The counterfeit's weight is different from that of a genuine coin, but you don't know if the counterfeit is heavier or lighter than a genuine coin. You also have an unusual balance (the picture shows a top view of it), with three pans instead of the usual two. Any number of coins can be placed in each of the three pans, and the balance will tilt toward the heavier pan or pans if the weights are unequal.
What is the minimum number of weighings needed to identify the counterfeit? (You may put coins in all three pans in a single weighing.)